Puzzle Solution Index

 INDEX OF PUZZLES AND SOLUTIONS 

Not familiar with KenKen puzzles?  Learn all about them at the

 KenKen Puzzle Introduction with Sherlock Holmes

Once you understand the puzzle you can see how some simple logical deduction can be applied to these problems:

Strategies For Deduction

Beginners can also check their calculations with a variety of free online...

KenKen Calculators for Factoring and Checking Possibilities

There are many free online versions of KenKen appearing under other names:
 

 By Many Other Names - KenKen Clones

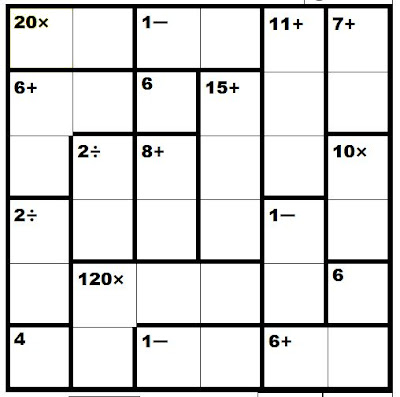
The solved puzzles:

These puzzles are listed in order from easiest to hardest (Puzzles 1-22).  

Puzzles 23 and 24 are 5x5 Puzzles with some interesting strategies used for their solution.

Puzzles 25-30 are a series of 5x5 normal difficulty puzzles that are excellent as a daily puzzle that is not too difficult or time consuming.  This series should be helpful for beginners who want some example solutions.

Puzzles 31 begins a series of 6x6 normal difficulty puzzles that are more challenging than the 5x5s. 

Puzzle 37 begins a series of 7x7 normal difficulty puzzles that increase the challenge.

• KenKen Puzzle Number 1 - 4x4


• KenKen Puzzle Number 2


• KenKen Puzzle Number 3


• KenKen Puzzle Number 4 - 5x5


• KenKen Puzzle Number 5


• KenKen Puzzle Number 6


• KenKen Puzzle Number 7 - 6x6


• KenKen Puzzle Number 8


• KenKen Puzzle Number 9


• KenKen Puzzle Number 10


• KenKen Puzzle Number 11


• KenKen Puzzle Number 12


• KenKen Puzzle Number 13 - 7x7


• KenKen Puzzle Number 14


• KenKen Puzzle Number 15 - 8x8


• KenKen Puzzle Number 16


• KenKen Puzzle Number 17 - 9x9


• KenKen Puzzle Number 18


• KenKen Puzzle Number 19


• KenKen Puzzle Number 20


• KenKen Puzzle Number 21


• KenKen Puzzle Number 22


• KenKen Puzzle Number 23: Interesting row summing


• KenKen Puzzle Number 24: Using a tree diagram 

 

Series of 5x5 Normal Level Puzzles

KenKen Puzzle Number 25: 5x5 Normal Level 

 

KenKen Puzzle Number 26: 5x5 Normal Level 

KenKen Puzzle Number 27: 5x5 Normal Level 

KenKen Puzzle Number 28: 5x5 Normal Level 

KenKen Puzzle Number 29: 5x5 Normal Level 

KenKen Puzzle Number 30: 5x5 Normal Level 

 

Series of 6x6 Normal Level Puzzles

KenKen Puzzle Number 31: 6x6 Normal Level 

KenKen Puzzle Number 32: 6x6 Normal Level

KenKen Puzzle Number 33: 6x6 Normal Level

KenKen Puzzle Number 34: 6x6 Normal Level  

KenKen Puzzle Number 35: 6x6 Normal Level

KenKen Puzzle Number 36: 6x6 Normal Level

Series of 7x7 Normal Level Puzzles

KenKen Puzzle Number 37: 7x7 Normal Level

KenKen Puzzle Number 38: 7x7 Normal Level

KenKen Puzzle Number 39: 7x7 Normal Level

KenKen Puzzle Number 40: 7x7 Normal Level

 KenKen Puzzle Number 41: 7x7 Normal Level

 KenKen Puzzle Number 42:7x7 Normal Level

 KenKen 6x6 Puzzles to Solve Without Using Scratch Paper

KenKen Calculators for Factoring and Checking Possibilities

A number of KenKen calculators and solvers are available online.  One useful one for beginners is:

CalcuDoku, Killer Sudoku and Kakuro calculator!


If you are a beginner and uneasy about factoring and establishing all possible cage solutions, then you can use this calculator to check to see if you have found all the possibilities for solving a cage.  We present the following example using the 48x cage from Problem 24 in this blog:

Above is the 48x cage.  For the 5x5 puzzle we see that four numbers are required between 1 and 5.  We also note that 3 doubles (or repeats of the same number) are possible:  on the two sets of diagonal squares as well as in the top and bottom squares.  This gives us enough information to plug into the above online calculator:

 Once we have plugged in these values, we press the calculate button and generate the answer:

 We see that this yields the same values that we established in the puzzle solution note:

We can look at another example, this time the 432x cage from Problem 22 in this blog:
              

This is a 9x9 puzzle and there is one possible double (or repeat of the same number) on the diagonal.  This is enough information to enter into the calculator:

 We press calculate and see the single result:

If we want to figure this out logically, without the calculator we start by breaking down 432 to its prime factors:

2 x 2 x 2 x 2 x 3 x 3 x 3

 We can see right away that 1 cannot be used, since 1x9x9 (the maximum number solution) is nowhere near 432!  We must now multiply some of these numbers together so that we are left with three numbers from the set 1 through 9. 

If we start at the low end and convert 2x2 into 4, we are left with 2x2x3x3x3 and there is no way to make two numbers under 10 from these factors.  If we start with 2x2x2=8 then we are left with 2x3x3x3 and we can see only one way to combine these into two numbers we can use:  2x3=6 and 3x3=9.  So we get the single possibility: 6x8x9.

We can work through a similar argument starting from 3 at the high end of these factors and arrive at the same conclusion: 6x8x9.


FACTORING HELP

For those who merely want help factoring a large number, there are a number of straight factoring calculators available.  Remember, however that 1 is always a factor and is often used.  One good calculator is:

Factoring Calculator

from Calculator.Net

If you plug in 432 from the above example you get the following result that breaks down the factors in several different ways.  For KenKen solvers, the PRIME FACTORS are the important ones since they give you the base to work out what possibilities can be used for any particular cage:

KenKen Puzzle Number 24: Using a tree diagram to clarify the logic

In this puzzle we employ a tree diagram for the first time to see the logical possibilities more clearly.  The 3 on top can combine with 2 or 4 in a 1- cage, and we can show how these possibilities combine with other numbers in a column to arrive at the column total of 15.  Check out the solution below to see this tree diagram at work!

 

 This is Inky #1 from Hard 5x5 Inkies by Krazydad, Volumn 3, Book 8

 And here is the Solution for Puzzle 24 of our Series

KenKen Puzzle Number 23: Interesting row summing logic in a hard 5x5 Puzzle

This 5x5 puzzle at the hard level offers some interesting row summing logic that lets us use the top row to crack the whole puzzle open.  Recall that for the 5x5 puzzles, each row and column must total 15 and contain the numbers: 1,2,3,4,5.

This is Inky #1 from Hard 5x5 Inkies by Krazydad, Volumn 3, Book 4

And here is the Solution for Puzzle 23 of our Series

KenKen Puzzle Number 22

 

This is Ink #2 from 

Mixed 9x9 Inkies by Krazydad, Volume 1, Book 1. 

  Here is the solution:

 Detailed Solution for Ink #2 from Mixed 9x9 Inkies

KenKen Puzzle Number 21

 

 This is KenKen Puzzle Number: 101048 (9x9  Easiest) from the 

Official KenKen Website

 

Here is the solution:

 Detailed Solution for KenKen Puzzle Number 21

By Many Other Names - KenKen Clones

KenKen Puzzles can be found on the Internet under many different names, usually to avoid copyright infringement on the KenKen name.  The following list is found on the official Calcudoku Website:

Calcudoku, Newdoku, Rekendoku, MathDoku, Kashikoku-Naru, KenKen, Kendoku, Sumdoku, Calkuro, K-Doku, Keen, NekNek, CanCan, Square Wisdom, Emono, Minuplu, LatinCalc, Yukendo, ArithmeGrid, Hitoshii, Inky, SquareLogic, TomTom

Many sites provide an option for solving their puzzles online as well as printing out puzzles to solve:

Calcudoku Puzzles Online

Mathdoku Puzzles Online

Web Kendoku Online 

Newdoku Online

Vegard Hanssen's Calcudoku Problems Online 

Caludoku provides an excellent page on basic and advanced solving techniques:  

Calcudoku Solving Techniques - Basic to Advanced

Calcudoku also provides an interesting online forum for players to discuss problems and exchange information:

Calcudoku Puzzle Forum 

The above forum contains an excellent thread on solving techniques: 

Calcudoku - Solving strategies and tips 

KenKen Puzzle Number 20

This is KenKen Puzzle Number: 101037 (9x9  Easiest) from the 

Official KenKen Website

Here is the solution:

 Detailed Solution for KenKen Puzzle Number 20

KenKen Puzzle Number 19

 

 
This is KenKen Puzzle Number: 101922 (9x9  Easy) from the 

Official KenKen Website

Here is the solution:

 Detailed Solution for KenKen Puzzle Number 19

KenKen Puzzle Number 18

This is KenKen Puzzle Number: 101434 (9x9  Easiest) from the 

Official KenKen Website

Here is the solution:

 Detailed Solution for KenKen Puzzle Number 18

KenKen Puzzle Number 17 - 9x9

We have finally got to the 9x9 puzzles that are the ultimate KenKen challenge.  Fortunately no new techniques are required yet.  The ones we documented are sufficient to solve this puzzle:

Strategies for Solving KenKen Puzzles 
Using Your Powers of Deduction

 

This is KenKen Puzzle Number: 100697 (9x9  Easiest) from the 

Official KenKen Website

And here is the detailed solution for our first 9x9 puzzle:

Detailed Solution for KenKen Puzzle Number 17

KenKen Puzzle Number 16

This is KenKen Puzzle Number 89363 Easy from the 

Official KenKen Website

And  here is the solution: 

Detailed Solution for Ken Ken Puzzle Number 16

KenKen Puzzle Number 15 - 8x8

Here is our first puzzle in the 8x8 size.  Lots more possibilities to narrow down, so even the easy 8x8 puzzles are often tricky.

This puzzle is from the Easiest 8x8 category on the 

Official KenKen Website

 The website has a great set of puzzles you can solve on line at many different levels of difficulty.

Here is the solution to this puzzle:

Detailed Solution of KenKen Puzzle Number 15

KenKen Puzzle Number 14

This puzzle is from 

 Mixed 7x7 Inkies by Krazydad,Volume 1, Book 1

Here is the solution:

Detailed Solution of KenKen Puzzle Number 14

KenKen Puzzle Number 13 - 7x7

This is our first 7x7 puzzle and comes from:

Mixed 7x7 Inkies by Krazydad, Volume 1, Book 1

Here is the solution:

Detailed Solution for KenKen Puzzle 13

KenKen Puzzle Number 12

This puzzle comes from 

Medium 6x6 Inkies by KrazyDad 
Volume 1 Book 1

Here is the solution:

Detailed Solution for KenKen Puzzle 12

Strategies for Deduction

Several different strategies are important for solving all KenKen puzzles using your powers of deduction. We will look at these strategies using examples from the puzzles solved in this blog.

Factoring 

We must be able to break or factor larger numbers down, as shown above, until you get to numbers you can use in KenKen puzzles.  This is useful when the cage you are looking at is one that involves multiplying.

When breaking a number down into its factors it is useful to include 1 and put the factors in order from smallest to largest.  This will help  you to determine in a systematic way what factors could combine together to go in the given cage.



Here is a 10x cage from a 5x5 KenKen Puzzle (Puzzle 6).    We must factor the number 10 down until we get some numbers we can use.    10 = 2x5 = 1x2x5   Note:  it is often helpful to remember that 1 is a factor of every number we will be using, and will sometimes be involved in the solution.   We can see that  1,2,5 must be the numbers that will be found in this cage.  No other factors are available.

Here is a 72x cage from a 6x6 puzzle 
(Puzzle 8).

We factor 72 first by splitting it in two and then factoring those numbers in turn until we reach numbers we can use, which for a 6x6 puzzle would be 1,2,3,4,5,6.

72 = 1x2x36 = 1x2x(2x18) = 1x2x2x2x9 = 1x2x2x2x(3x3). 

Here we have five factors but only three squares in the cage, so we will have to multiply some of the factors together again to get other possibilities for example as follows: 3x(2x2)x(2x3) giving 3, 2x2=4 and 2x3=6 or 3,4,6.  One can quickly see that no other way of combining these factors into three numbers will give us anything that works in a 6x6 puzzle.

Often you will find that there is in fact only one set of numbers that works, so this strategy can be extremely useful.

 Here is a 25x cage from a 6x6 puzzle (Puzzle 8)





We know that 25=1x5x5. If the cage was in a row or column, as with the above two, then we know we would not be able to solve it, since that would involve putting two 5’s in the same row or column. But in this case we are able to use the two 5’s by placing them on a diagonal to one another, and the 1 in the other square. This is clearly the only way to solve this cage. 

Doubles, Triples and Quads

These are techniques that should be familiar to avid Sudoku and Kakuro solvers.

 Here is an example of a column double in a 5x5 puzzle. There are no other number possibilities in a 5x5 problem. For a 6x6 you would also have to look at 2x6. A column double means that you know what are in those two squares and that all the other squares in the column cannot contain either of these numbers. Same works for doubles in a row as opposed to a column.

Here is an example of a column triple from a 6x6 puzzle. We would notice that 15 only has three factors: 1x3x5. Even though we might not know which squares the numbers go in, a triple further narrows down the possibilities for the other squares in the row or column.



Quads are rarer than doubles and triples, but the same principles apply, only for four squares in a row or column.


 Adding by column or row

 Like a Suduko puzzle, all the rows and columns must contain all of the same set of numbers without any duplicates.

This means that if you sum up the squares in any row and column you will get the same total. For a 6x6 puzzle this total would be 1+2+3+4+5+6 = 21.

This information can be used in a variety of powerful ways to sum part of a row or column to deduce numbers from another part:

If we look at this column (from Puzzle 7) we see two 5+ cages which will total 10. The first column number in the 30x cage is 5 or 6. If we add these together we get two possible totals: 15 or 16. Therefore the bottom number in the first column must be a 21-15=6 or 21-16=5, but since 5 is not a possibility there, then the number must be 6









Multiplying by column or row

 



In this example from a 5x5 puzzle (from Puzzle 5) we can see that the numbers in the right hand column must be 1,2,3,4,5. We can therefore figure out the number at the bottom of the left hand column by multiplying together the numbers in the right hand column and dividing this product into 600:

600 / (1x2x3x4x5) = 600/120 = 5.





“Too Big for Your Britches” Strategy

In this 6+ cage (from Puzzle 11) we can see that the bottom number cannot be 5 because 5 is TOO BIG to leave any numbers for the other two squares!







“Little Britches” Strategy

 In the row above (from Puzzle 19, 9x9) we see a variation of the "Too Big For Your Britches" scenario which we will call "Little Britches".  A scan of the row shows that the number 1 is not shown anywhere as a possibility.  We look at the 11+ cage and see that 1 is TOO SMALL to use in that cage, and therefore must go in the empty square in the middle of the row.

 
Scanning Rows and Columns

Sometimes you cannot see the forest for the trees.  When you seem to be at a dead end, it sometimes helps to step back and look at the larger picture:

In scanning the row above from a 5x5 puzzle (Puzzle 5) you suddenly notice that the number 4 does not appear, and therefore MUST be the number in the last, empty square!

Let us now look at the following row from a 7x7 puzzle 
(Puzzle 14).  

We can see that there is no 7 among the possibilities shown.  Therefore we must conclude that 7 is in one of the two empty squares on the left.  If a 7 is in one of these squares then we may also conclude that a 6 must be in the other to solve the 1- cage.

The column here to the left (from 7x7 Puzzle 14) has no 6 showing and so we may conclude that 6 is in one of the two empty bottom squares.

















In Puzzle Number 25 of this blog, we seem to have little to work with at first:

 
But look at the straight line 5 + cage in the second
last row. We know that the 5 cannot be in the 5+ cage and so
must be in one of the other three squares of that row. We know
that all the numbers in the 24x cage must multiply together to get
24, but 5 is not a factor of 24. 5 is a factor of 10,15,20,25,30, etc.
and the pattern is clear and does not include numbers like 24.
There is now only one square left in that row for the 5 and so we have found our first number for this puzzle!

Accentuate the Negative

 Sometimes when possibilities are numerous, it is better to show what is IMPOSSIBLE rather than the possibilities:

For the above row from a 6x6 puzzle (Puzzle 12) we can plainly see that the number 4 is IMPOSSIBLE for the 4- cage.  With this information added, we can scan the row and see that there is indeed only one square in the row that could be a 4.

X-Wing 

This is a strategy that is also used in Sudoku puzzles.  It usually involves having the same number in doubles on two columns or two rows.   Here is an example from  Puzzle 8:

 We notice that in the top two rows there are doubles which both contain the number 4.  This is an X-Wing configuration and means that the number 4 must be in one of these squares in both the fourth and fifth columns.  Therefore we may conclude that no other square in these two columns can contain a 4.  For example we have 2,4 as initial possibilities for the 6x cage in the fifth column, but from the X-Wing we know that the 4 must be in one of the two squares at the top of the column and therefore can be rejected as a possibility in the 6x cage.  Of course we also know that 4 is not a factor of 6, and so we have two separate ways to reject the 4 in the 6x cage.

The above X-Wing example has adjacent squares, but really any doubles on any two columns and rows can be X-Wings.  In the example below (from Puzzle 10) we see a 1,2 double in the top row and a 2,3 double on the bottom row, and together these form an X-Wing that shows 2 can only be in the top or bottom squares of the third and fourth columns.

 

KenKen Puzzle Number 11

 

This puzzle is Inky 3 from 

 Medium 6x6 Inkies by Krazydad, Volume 1, Book 1

Here is the solution:

 Detailed Solution for KenKen Puzzle Number 11

KenKen Puzzle Number 10

This is Ken Ken Puzzle Number: 40091 
from the official Ken Ken Website.

Here is the solution: 

Detailed Solution of KenKen Puzzle 10

KenKen Puzzle Number 9

This is Ken Ken Puzzle Number:  96009 
from the Official Ken Ken Website

And here is the solution:  

Detailed Solution for KenKen Puzzle 9